December 2024
Welcome to EFM's December Newsletter!
It is essential that every caregiver in the world reads books and does math with their young children!
EFM believes in every child’s mathematical right to equity, opportunity, and personal fulfillment.
News
Two Mobile Apps – EFM and ESM. “Early Family Math” is available for free download from both app stores. It has full versions in English, Spanish, and Simplified Chinese. It has partial versions in French and Arabic – these languages, along with versions in Portuguese and Turkish, are improving every week.
We have begun development of our Early School Math (ESM) mobile app in earnest. This app is for teachers of early math who want some quick and easy mentoring or additional resources right at their fingertips on their phone. It is being designed with the needs of low-resource environments in mind, though it will eventually have lots of material for all environments. We now have a very early prototype which you can take for a test drive in a browser window – when you open this link, narrow the browser window to get the mobile phone feel. It is far from finished, and we plan on adding many improvements and many resources. If you try it out and have feedback or are intrigued by the possibilities, please contact me and let me know your thoughts – we welcome your thoughts and involvement at any level.
Celebrating EFM Volunteers
Everyone at Early Family Math is an unpaid volunteer. Everyone. It is such a pleasure to be working side by side with all these wonderful people who believe in EFM’s mission. For the last two months I have been recognizing some of our volunteers, and I would like to continue that tradition this month.
Elita
Elita has been with EFM from the beginning. She was a junior in high school when she started many years ago. She did our earliest translations into Chinese, she wrote up our successful 501(c)(3) application without using a lawyer, and she is the corporate secretary on our board of directors.
Japanese – Yuri, Makiko, Chisa, Maho, Mina, Hana, and Kyoko
These seven have done the hard work of translating the 58 EFM storybooks. They have started work on the 5 Stages and the EFM decks of playing cards.
Turkish – Büşra
She comes from Turkey and holds an advanced degree in math education. She is motivated to translate the EFM Stages so that Turkish families can benefit from our program.
Fitting Shapes Together
I vividly remember walking with my wife around the block one evening when we came across a father walking with a child in a stroller. The father had just been stunned to have his child point at the stop sign and say “octagon.” He had no idea his child knew the word for this shape and could recognize the shape of a stop sign.
Knowing the basic properties and names of shapes is important. These ideas are the first steps along a journey of exploration into geometry. You can reinforce these basic ideas with games involving describing and recognizing shapes, such as I Spy and Treasure Hunt.
However, once the basics are mastered, it’s time to start playing with activities that take us more deeply into this material.
Compare, Contrast, and Group
Comparing, contrasting, and grouping shapes are ways to thoughtfully explore what makes each shape special. The four-sided shapes in particular are a rich playground for that kind of questioning: what makes a square, a rhombus, a rectangle, a diamond, a parallelogram, a dart, and a kite different from each other, and what do pairs of them have in common? Which of those is always one of the others – e.g. is every square a rhombus and is every rhombus a square?
The exercise of separating a set of shapes into groups that have a common property trains the eyes to see properties in fresh ways, and it is great environment for a child to communicate reasoning. A popular version of this is Which One Doesn’t Belong? If you have four things, find the one that doesn’t have a property that the other three share. Your child sees the world very differently than you do, so be open to unusual answers and listen carefully to their explanations. Similar to WODB is Who’s the Spy? This activity comes from Think Square and challenges you to find the item that has the most differences despite its trying to blend in with the others.
Fitting Shapes Together
All of which brings me to the focus of this newsletter: playing with how shapes fit together is an engaging way to learn about shapes at a deeper level. You can tell someone about the sizes of angles and how it takes 360 degrees (or “one full turn”) worth of angles to fill in a corner where pieces meet, but that understanding is nothing compared to moving around polygons and seeing how they fit together.
Jigsaw Puzzles
Jigsaw puzzles are a simple, nontechnical way to play with fitting shapes together. Though the shapes are usually not the traditional geometry shapes, there is still lots of training of the eyes involved. Also, describing the color and shape for a piece you are looking for builds geometry vocabulary and understanding.
You can make a jigsaw puzzle in an instant by taking any colorful box top and cutting it into interestingly-shaped pieces.
Pattern Blocks – Free form and Challenges
Wooden pattern blocks are a fantastic toy for experimenting with how polygons work together. The wooden pieces usually have a wonderful feeling in the hand, and the coloring of the pieces provides an opportunity to make visually interesting design. There are many sources of these around the internet, and Math for Love has two sets of these pattern blocks that have particularly interesting sets of pieces.
Here is a free form design (that is also a semiregular tiling) my son made many years ago.
Making pleasing free form designs with pattern blocks may be an unstructured activity; however, a child learns a great deal in the process of getting the pieces to work out in ways that they want.
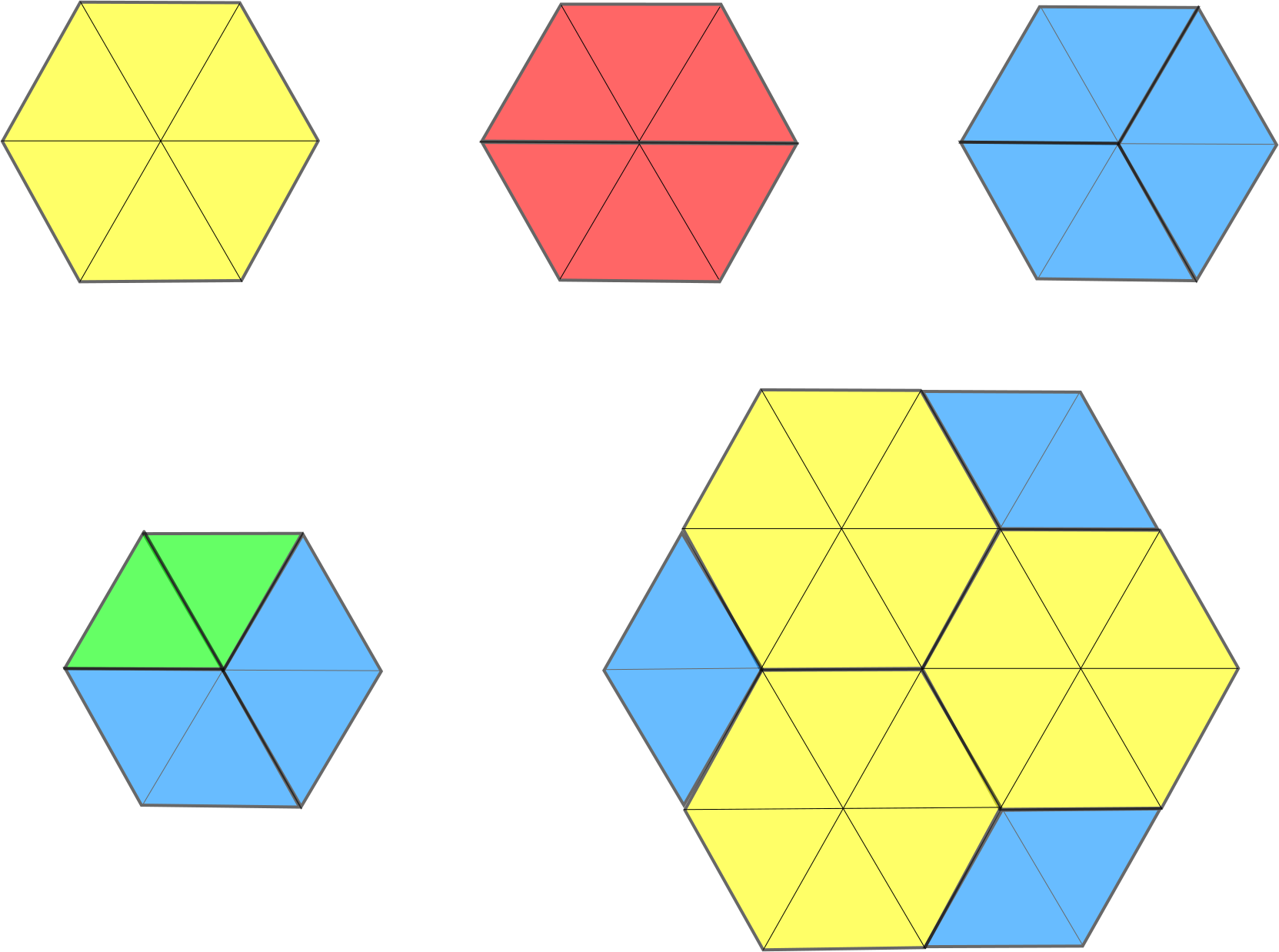
There are also many structured challenges associated with pattern blocks. Two popular challenges are seeing all the ways you can use different counts and types of pattern blocks to make a larger triangle or hexagon.
Pattern Blocks – Tilings
Creating tilings provides some interesting challenges. Regular tilings use one type of regular polygon to make an endless repeating pattern with it (e.g. honeycomb). Investigate with your child which regular polygons work to make one of the three regular tilings.
The world of the eleven semiregular tilings has more variety and more interesting patterns. A semiregular tiling is one that uses regular polygons where every point in the tiling where three or more polygons meet looks the same (if you were a little bug at that point). The son’s tiling example earlier in this newsletter is an example of a semiregular tiling. Play with your child to find examples where a set of polygons can fill space with a repeating pattern with no holes.
By the way, if you would like to avoid large numbers up to 360, instead of describing angles in degrees, describe them as a fraction of a full turn. Using this description, the angles for regular polygons are: triangles – 1/6 turn, squares – quarter turn, pentagons – 3/10 turn, hexagons – 1/3 turn, and octagons – 3/8 turn. In general, if n is the number of sides, the angle will be (½ – 1/n) turns.
Puzzles with a set of shapes
EFM has several investigations where one kind of shape is used repeatedly to fill in a bigger shape. One example is the puzzle of seeing what all the possible numbers of squares are that can be put together to form a bigger square. One can ask the same question about the number of triangles that can be put in a bigger triangle.
Both puzzles have some initial easy answers produced by scaling: you can get 1 from 1 by 1, 4 from 2 by 2, 9 from 3 by 3, and so on. The next steps after seeing that the square numbers all work is where the fun starts. I visited this topic in the EFM January, 2023 newsletter.
To get you started with the non-square numbers, here are examples for putting 1, 4, or 7 squares in a bigger square.
Breaking Shapes Apart
Some shape puzzles are oriented towards deconstruction rather than construction. While these may not teach your child new things about angles, it will help them see where unexpected shapes can fill in the gaps.
Here is an example from the “Finding the Pieces” EFM Puzzles of the Week and the playing card decks. In this puzzle, the challenge was to take the black outline of the house and fill it with standard shapes (shown in red).
Wrapping Up
I hope you enjoyed these ideas for playing with shapes. I also hope that you and those you care about have a safe and wonderful holiday season. I wish you all a Happy New Year!
If you have any questions or comments, please send them our way! We would enjoy the opportunity to chat with you. Also, if you are interested in collaborating with us or supporting us in any fashion, we would love to talk with you about ways we can work together!
December 18, 2024
Chris Wright
Chris@EarlyFamilyMath.org
Twitter | Facebook | Instagram
Early Family Math is a California 501(c)(3) nonprofit corporation, #87-4441486.